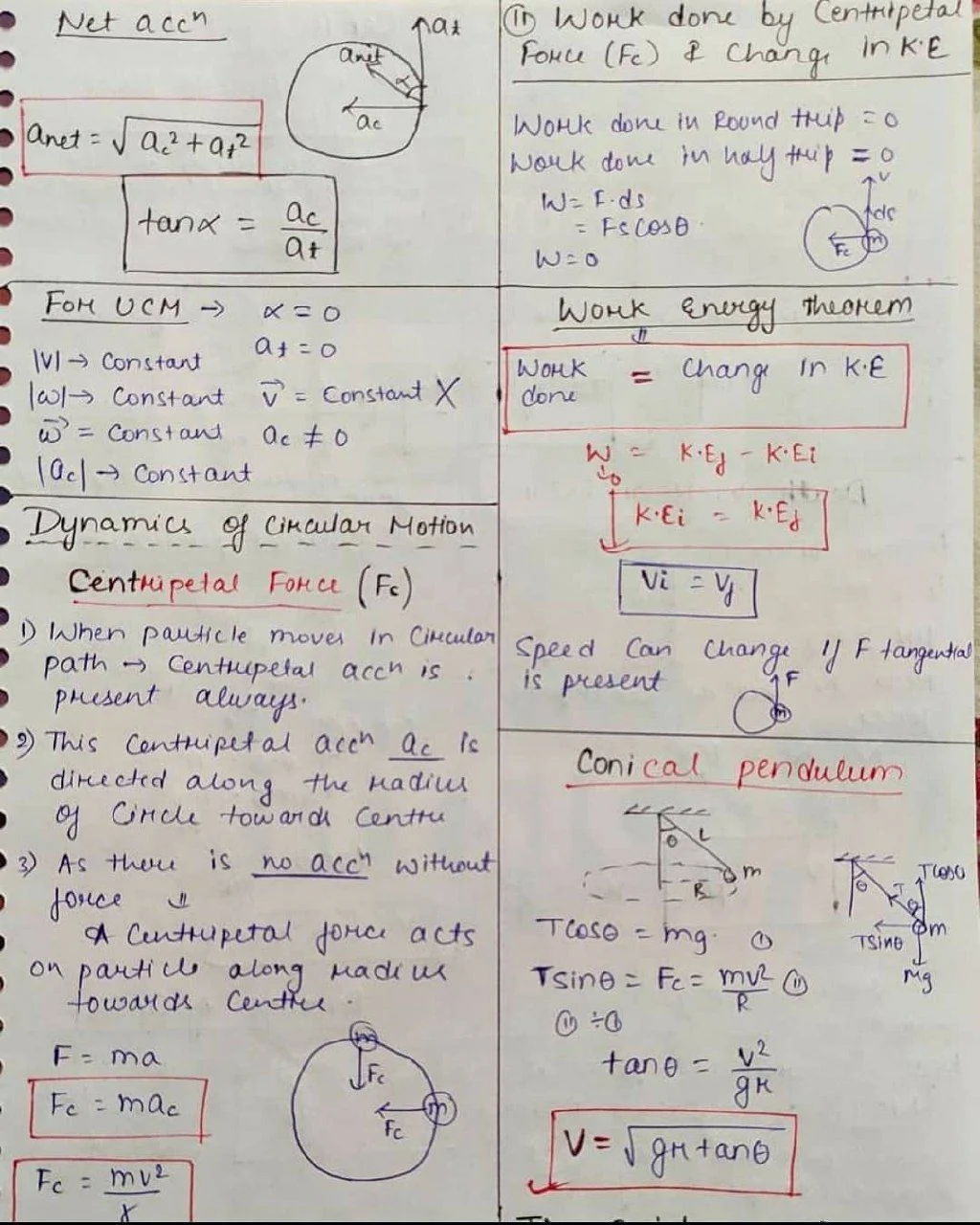
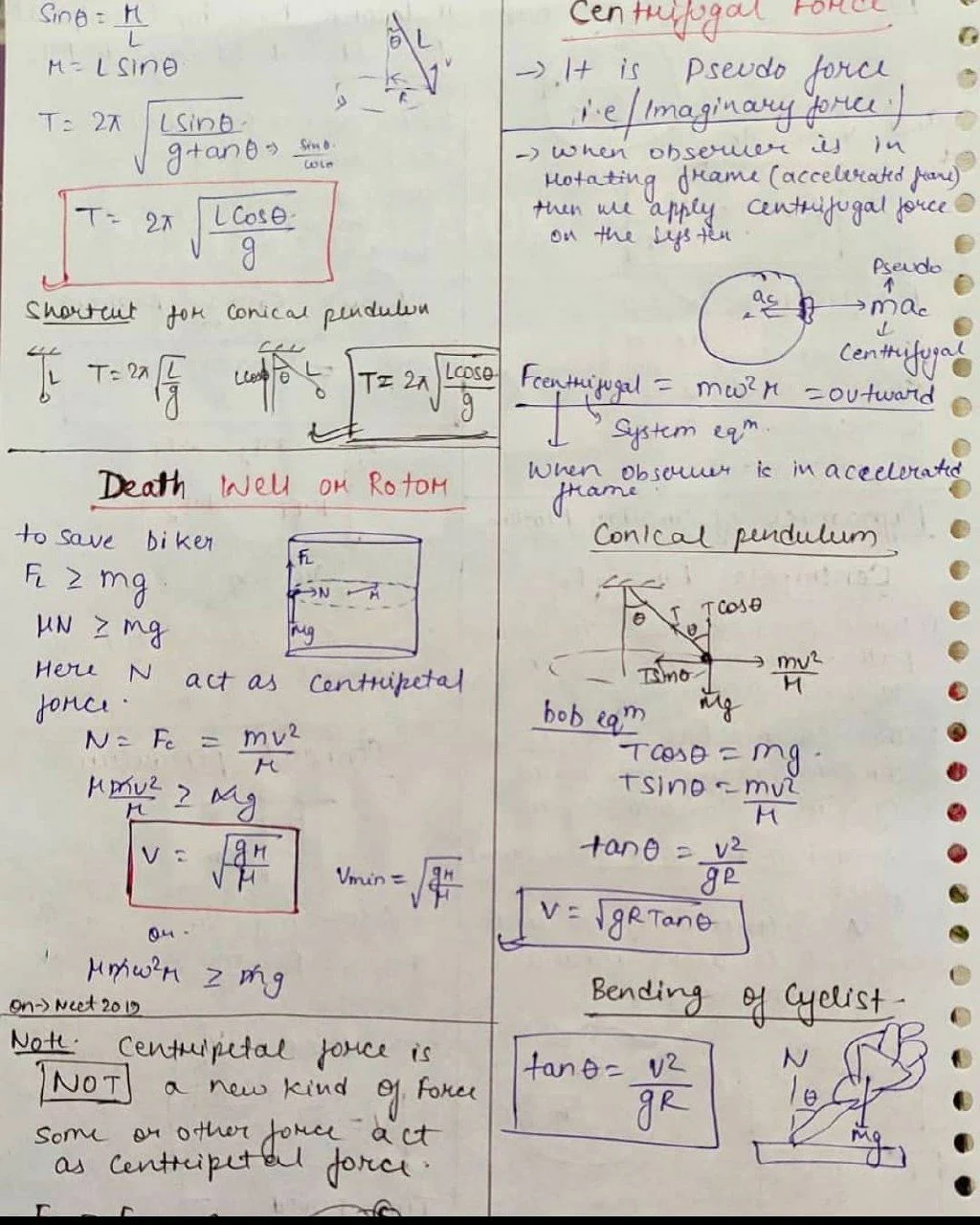
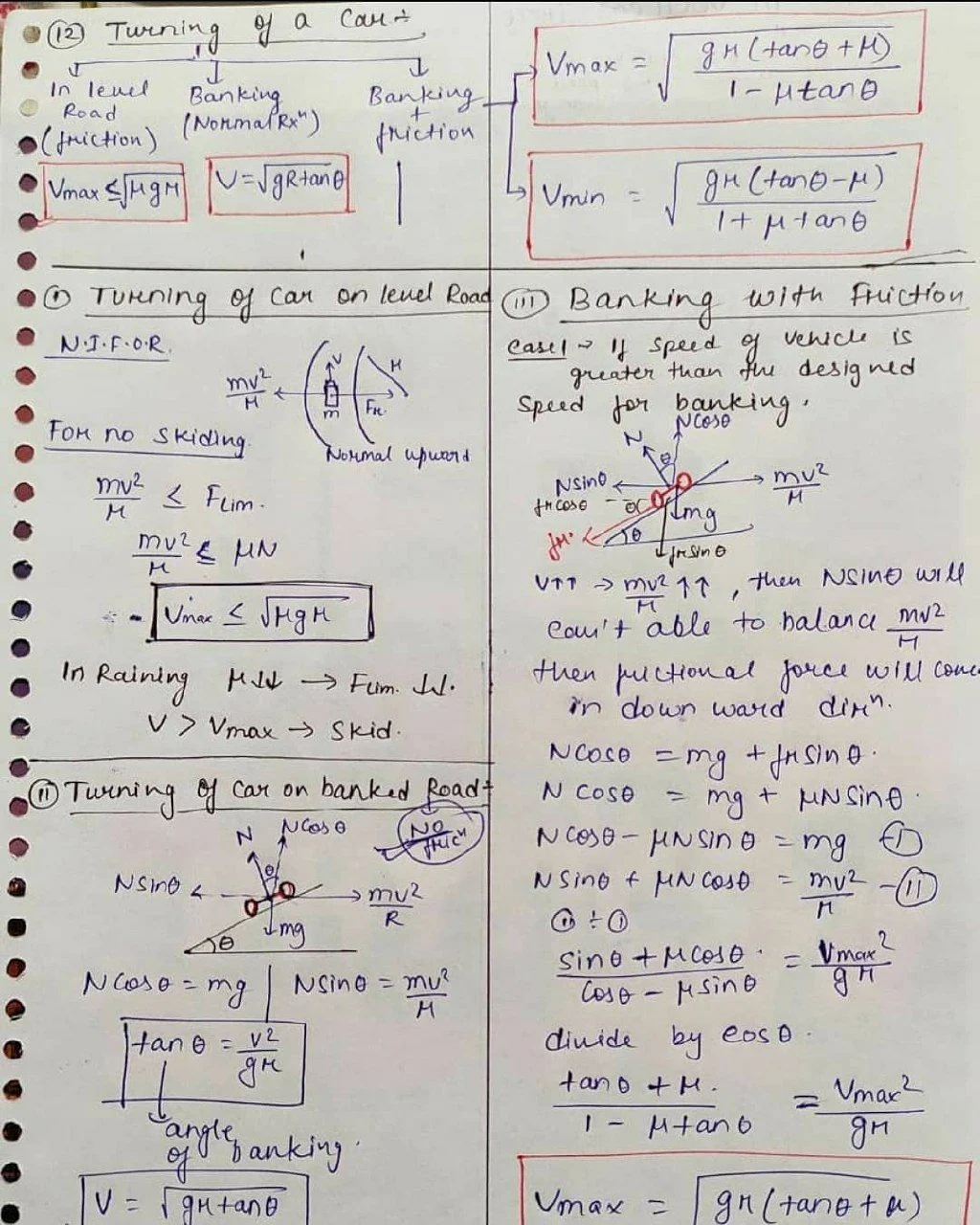
Circular motion is a fundamental concept in physics that describes the movement of an object along a circular path. This guide delves into the fascinating world of circular motion, exploring its definition, different types, real-world examples, practical applications, and essential formulas.
Definition
Circular motion refers to the continuous movement of an object around a fixed point in a circular path. While the object's speed (magnitude) might remain constant, its direction keeps changing continuously. This constant change in direction necessitates the concept of acceleration even though the speed stays the same.
Types of Circular Motion
Circular motion can be categorized into two primary types:
⦿ Uniform Circular Motion: In this type of motion, the object maintains a constant speed throughout its circular path. The magnitude of its velocity (speed and direction) remains constant, even though the direction is continuously changing.
⦿ Non-uniform Circular Motion: Here, the object's speed keeps varying along its circular path. This variation in speed results in a change in both the magnitude and direction of its velocity.
Examples of Circular Motion
Numerous everyday phenomena involve circular motion:
⦿ A car turning on a curved track.⦿ A child on a swing set.
⦿ A satellite orbiting the Earth.
⦿ The blades of a rotating ceiling fan.
⦿ The gears in a mechanical watch.
These examples showcase the diverse applications of circular motion in our world.
Applications of Circular Motion
The understanding of circular motion plays a crucial role in various scientific and engineering fields:
⦿ Satellite communication: Satellites rely on circular motion to stay in orbit around the Earth, enabling communication technologies.
⦿ Amusement park rides: The design of Ferris wheels, roller coasters, and other rides utilizes principles of circular motion to create thrilling experiences.
⦿ Centrifuges: These machines employ circular motion to separate components of different densities in mixtures.
⦿ Circular saws and grinding machines: Cutting tools leverage circular motion for efficient operation.
Understanding circular motion empowers engineers to design and develop various technologies that impact our daily lives.
Essential Formulas for Circular Motion
⦿ Circumference (c): c = 2πr (where r is the radius of the circle and π is a mathematical constant approximately equal to 3.14)
⦿ Angular speed (ω): ω = 2πf (where f is the frequency, the number of revolutions per unit time)
⦿ Period (T): T = 1/f (the time taken for one complete revolution)
⦿ Linear speed (v): v = ωr
⦿ Centripetal acceleration (ac): ac = v²/r (the inward acceleration acting on the object due to its circular motion)
These formulas provide a quantitative framework for analyzing and solving problems related to circular motion.
By comprehending the concepts and applications of circular motion, we gain a deeper appreciation for the diverse motions occurring in our world, from the grand celestial dance of satellites to the simple swing of a child on a playground.
FAQs about Circular Motion
Q: What's the difference between speed and velocity in circular motion?
A: Speed refers to the magnitude of an object's movement, how fast it's traveling. In circular motion, even with constant speed, the object's direction is continuously changing. Velocity, on the other hand, considers both speed and direction. So, while the speed might be constant, the velocity keeps changing due to the directional shifts.
Q: Why does an object in circular motion need acceleration even if its speed is constant?
A: Even though the speed (magnitude) stays constant in uniform circular motion, the direction keeps changing. This change in direction necessitates acceleration. This inward acceleration, called centripetal acceleration, acts towards the center of the circular path and is responsible for constantly changing the object's direction.
Q: How can we change the centripetal acceleration of an object in circular motion?
A: There are two main ways to influence the centripetal acceleration:
⦿ Changing the speed (v): If you increase the speed of the object while maintaining the same circular path, the centripetal acceleration will also increase (proportional to the square of the speed). Conversely, decreasing the speed reduces the centripetal acceleration.
⦿ Changing the radius (r): For a constant speed, increasing the radius of the circular path reduces the centripetal acceleration (inversely proportional to the radius). On the other hand, decreasing the radius increases the centripetal acceleration.
Q: What happens if the force providing the centripetal acceleration is suddenly removed?
A: If the force responsible for the centripetal acceleration disappears, the object will no longer be able to follow the circular path. It will move along a straight line tangent to the circle at the point where the force vanished. Imagine a stone tied to a string; when you whirl it around and let go of the string, the stone flies off tangentially due to the absence of the centripetal force (the tension in the string).
Q: Are there any real-world examples of non-uniform circular motion?
A: Yes, many real-world scenarios involve non-uniform circular motion. Consider a car entering a turn on a racetrack. As it enters the turn, its speed might decrease slightly due to factors like friction. This decrease in speed signifies non-uniform circular motion. Similarly, a frisbee thrown at an angle experiences non-uniform circular motion as its speed reduces due to air resistance.



![Circular Motion - Physics Short Handwritten Notes [PDF]📚 Circular Motion - Physics Short Handwritten Notes [PDF]📚](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEi8C8nHQtOlNURdLrhOTd4QnHGtQfE8zKkdInsfOJj6vNdr78i3Almr-QEf-jMFchcJnr3wGhQMwNVIBMw2YIPGVwv47j8YWDv74uZAQ8C008xFdkwMjWk1iz-KaPsvliK3_1VY-LZqT5ygS0eJGr5uE4P2b8Qpax-fRm_JCue-uHoNtdSy4IArTZSxQaU/s16000-rw/CircularMotion%20Physics%20Short%20Notes-images-1.jpg)
![Circular Motion - Physics Short Handwritten Notes [PDF]📚 Circular Motion - Physics Short Handwritten Notes [PDF]📚](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEj7ZMBzVYmNdfa1-hvRBsrEMbHlQJqvPurHDpSF4RFyQqWffrsjDLbtw1cYC2eebxz5uvz6vhHR0wZpqbvxNhLc5zsCttWcURWDWW-sAcMpv9XpbvzkyfEo_c-JoD8n4BnKBHb_6BG9FJcMRcg61Zls3Ch1Qt2Rn_6QsiHxe5jC3saDYgRBPv_DdygqHEw/s16000-rw/CircularMotion%20Physics%20Short%20Notes-images-2.jpg)